Various important events and important contributions were made in the period 500 BC – 400 AD which we call it as Post Vedic period. Religions like Buddhism, Jainism were formed; first Indian scripts- Brahmi scripts (derived from Kharoshti scripts) were recorded and which led the foundation for Devanagari numbers which ultimately led to the numbers which we follow today, epics like Ramayana and Mahabharata were written, complete treatise on Sanskrit grammar which was prepared by Panini and is even followed today, etc.

[wptabs style=”wpui-narrow” effect=”slide” mode=”horizontal”][wptabtitle] Jaina & Buddhist Mathematics[/wptabtitle] [wptabcontent] Jaina mathematics is one of the least understood chapters of Indian mathematics, mainly because of the scarcity of the work. Jain religion was formed by Varadhamana (Mahavira) around in the period 570 BC. Jaina mathematics is termed as mathematics which was followed by those who followed Jainism and was seen in the period 400 BC – 200 AD. Jaina mathematics could be seen written in various Sutras like Surya Prajnapati, Bhagabati Sutra, Sthananga Sutra, Jambudvipa Prajnapti , Vaishali Ganit, Uttaradhayyan Sutra, Anuyoga Dwara Sutra, Tiloyapannatti, etc. Many topics on mathematics were discussed in Sthananga Sutra: arithmatic operations like multiplication, division, subtraction, addition; Number Theory; Geometry;Mensuration of solid objects like sphere, cylinders,etc; fractions; Solving simple, quadratic, cubic, biquadratic equations, laws of indices and Permutation & Combinations. Like people from Vedic period, Jaina mathematicians were also interested in cosmology and large numbers.

Jain cosmology says universe, as an uncreated entity, existing since infinity,the shape of the universe as similar to a man standing with legs apart and arm resting on his waist. This Universe, according to Jainism, is narrow at the top, broad at the middle and once again becomes broad at the bottom.

Infinty:

This brings the topic of infinity. They classified infinity in 5 types: infinity in 1 direction, in 2 directions, in an area, everywhere and perpetually infinite. They were able to calculate that cosmology contains a time period of 2588 years= a number with 178 digits which is a very large number and that many number of years calculated as the time period of cosmology which can be considered almost infinity.
Laws of Indices:

In their work, laws of indices were also seen. Eg: The first square root multiplied by the second square root is the cube of the second square root. = (√a).(√√a) = (√√a)³.
Classification of Numbers:

Jaina mathematicians classified numbers in 3 groups: Enumerable (that can be counted), Innumerable (large numbers to count) and Infinite (difficult to count).
Geometry:

Geometry: Various solid and general geometrical figures and terms were used like circle, arc, chord, ellipse, sphere, cylinder, etc. They calculated value of pi as sqrt of 10.

Pingala:

Pingala was born in the 4th century of BC and was the younger brother of Panini. He used the concepts of mathematics in his work Chandahsastra, a Sanskrit poetry.

Panini born around 6th century BC is well known for his formulation of rules, grammar, semantics of Sanskrit language written in his treatise ‘Ashtadhyayi’. Panini’s scientific theory of Sanskrit is marked as the beginning of Classical era of Sanskrit and his work was so complete and correct that it is followed still today.

In Pingala’s work Chandahsastra following things can be seen:

First ever description of Binary number system(which is the language of computers) consisting of patterns which are in terms of long and short syllables.
Binomial theorem
Pascal’s triangle
Fibonacci series

He followed the Patterns of long and short (modern patterns are with 0’s and 1’s) Example: In his pattern of 4 syllables, he used them as

Syllables	Possibilities/Patterns	No. of Patterns	Combinations
4 short	SSSS	1	⁴C₀
3 short & 1 long	SSSL, SSLS, SLSS, LSSS	4	⁴C₁
2 short & 2 long	SSLL, LLSS, SLSL, LSSL, LSLS, SLLS	6	⁴C₂
1 short & 3 long	SLLL, LSLL, LLSL, LLLS	4	⁴C₃
4 long	LLLL	1	⁴C₀

Column 2 in modern binary system is written in terms of 0’s and 1’s.
Patterns in column 3 are same as that of coefficients of terms with a power of 4[Binomial Distribution: (a+b)⁴].
Column 4 shows the knowledge of Combinations Theory.

Likewise, various combinations of patterns can be seen in his work. Again these patterns were not for pure mathematical purpose but were used in writing Sanskrit poems. The patterns were named separately and were used as words in poems and these patterns are called as metres/rhythms in terms of poems. Eg: LSS was called as Bha, SSL as Sa, L as Gu, S as La, SLS as Ja, etc.

Fibonacci Series:

Fibonacci series can also be seen in these patterns. Taking 1 unit for S and 2 units for L we have.

Units	Patterns	No. of Patterns
1	S	1
2	SS, L	2
3	SSS, SL, LS	3
4	SSSS, SSL, SLS, LSS, LL	5
5	SSSSS, SSSL, SSLS, SLSS, LSSS, LLS,SLL, LSL	8
6	SSSSSS, SSSSL, LSSSS, SLSSS, SSLSS, SSSLS, LLSS, SSLL, LSSL, LLL, SLSL, LSLS, SLLS	13

Last column shows the Fibonacci series pattern.

These patterns were discovered by Gopala and Hemachandra from the poems of Sanskrit around 1000 years later and are named them as Hemachandra-Gopala pattern of numbers. But these numbers today are known as Fibonacci series as these numbers were introduced to the western world by Fibonacci, born around 50 years after them, through his book “Liber Abaci”.

[/wptabcontent] [wptabtitle] Numerals & Manuscripts[/wptabtitle] [wptabcontent]

Brahmi Numerals:

Brahmi numerals were the ancient Indian numeral system which were followed between 300 BC and 500 AD. This numeral system was based on decimal system (base 10) which we follow today. Brahmi numerals were modified many times in this period and finally arriving at the numerals which we use today. Brahmi numerals are the ancestors of Hindu/Hindu-Arabic numerals. Brahmi numerals are derived from Kharoshti Numerals which were present in the period between 400 and 300 BC. In kharoshti numerals, 1/2/3 were indicated by vertical lines like that of Roman while in Brahmi numerals, they were indicated by horizontal lines.

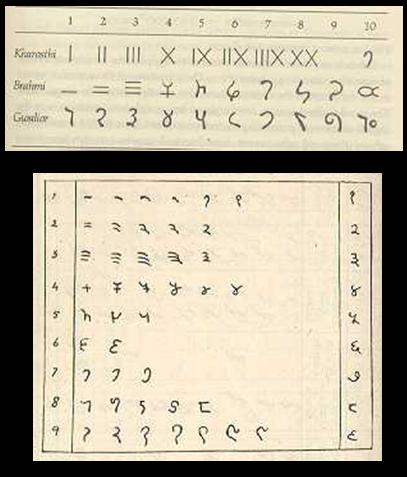
1BrahmiNumerals

2nd Image shows the Transition of Brahmi Numerals over the years.Zero was not yet invented.

Bakhashali Manuscript:

This manuscript is the ancient Indian manuscript written on birch bark which was found near the village of Bakhshali which is in Pakistan today. Not all barks are found, some are still missing while many are destroyed/scrapped. Bakhshali manuscript is considered as the oldest Ancient Indian Mathematical manuscript. These manuscripts are from 200 BC – 400 AD. (End date is not known exactly but it is surely before Aryabhatta’s time as in the scripts 0 was denoted by Dot). 2BhakshaliNumerals Mathematics found in Bakhshali Scripts:

Arithmetic operations. But the words were used instead of symbols. Eg ‘bha’ was used to indicate division
Fractions
Technique for calculating square roots
Solving Equations
Negative numbers

Fractions:

Fractions were not so different to that used today, written with one number below the other. No line appears between the numbers as we would write today. Another unusual feature is, the sign + was placed after a number to indicate -(subtraction)/negative number.

Squareroot Formula:

They used formula formula to calculate square root and surprisingly it was very close to accuracy. √Q = √(A² + b) = A + b/2A – (b/2A)²/[2(A + b/2A)] Where A = square root of perfect square just less than that Q, b = Q-A.

Examples:

√41
Q = 41, then A = 6, b = 5
Bakhshali formula gives 6.403138528.
Correct answer is 6.403124237
Here 8 decimal values are correct.

√339009

Bakhshali formula gives 582.2447938796899
Correct answer is 582.2447938796876
Here 11 decimal values are correct.

[/wptabcontent] [/wptabs]

Post Vedic Period

Infinty:

Laws of Indices:

Classification of Numbers:

Geometry: